sunskytalk.blogg.se - Calculate mac for center of gravity

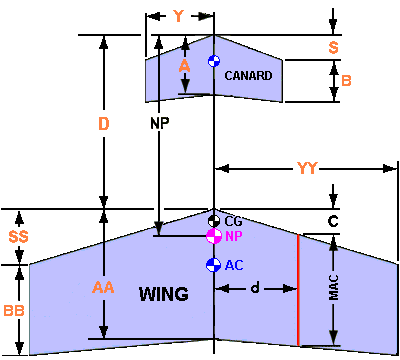
This initial estimate was necessary as a starting value for the iteration to be carried out here and in order to have tail parameters available for calculating mass and center of gravity. The CG of the airplane is located at 27.4 percent MAC. The tail lever arms were estimated as a percentage of the length of the fuselage. MAC can be quite complicated to determine, particularly if the. calculated merely with the aid of tail volume coefficients. I left the sphere in the model to show that the LCS is at the same exact location.įC018_centerofmass_LCS_01.png (39.14 KiB) Viewed 2529 times centreofmass2_normandc1. aircraft empty weight and empty weight centre of gravity position, together with suitable.

Under the Attachment mode list, select Inertial CS.

Press the Create a new local coordinate system icon.

From the Model tree (not the 3D view!), select Pad.

I had to fiddle with the tool to make sure the selected reference switched to the whole Pad "Solid". I found it quite tricky to reference the Pad rather than the selected face. atpl online practise tests question & answers in c-g-calculations, Calculate the centre of gravity in MAC (mean aerodynamic chord) with following.

If you need to know the of the Mean Aerodynamic Chord (MAC) for a given position of the CG you should use the Mean Aerodynamic Chord Form. So you can attach it with the Inertial CS mode, and there is no need for typing of expressions! This form will calculate the position of a Center of Gravity (CG) on a model airplane wing as measured from the leading edge of the Root Chord. Aerodynamic Center (AC), Mean Aerodynamic Chord (MAC), Center of Gravity (CG), Neutral Point (NP) and Wing Area. This margin for stability is called Static Margin. For longitudinal stability the CG is placed 5 to 15 of MAC in front of the NP. The CG value is always measured from the leading edge (LE) in the center of the main wing. What's more, since it's a datum tool, it uses the attachment system. The Center of Gravity (CG) is the point where the aircraft's weight is balanced. I have no clue what's the status of the 0.18 release for macOS, nor if the 0.19_pre builds are usable? Edit: I just realized that chrisond is still using 0.17, this method won't be available. Using a Part Sphere to display the location of the center of mass is a good idea, but here's another one: since you have a PartDesign Body, you can use the new (in 0.18) PartDesign CoordinateSystem. I have attached a simple demonstration file, where I have a Part WB default Sphere placed at the x,y and z, co-ordinates